Quote:
Originally Posted by Neapolitan
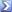
If you understand that when the "/" line is used, only divided the number underneath divides the number on top, in this case 6/2 means 6 divided by 2, (only 2 not the rest of the equation),
6/2 = 6÷2
|
Again, this adds nothing to the argument, it simply states it differently. Here is where we see to be coming to a disagreement at:
You say that multiplication and division both bear equal precedence between one another, therefore, in a complex equation involving both multiplication and division, one should simply operate from left to right.
My disagreement, which I supported with numerous and equally credible links, says that multiplication ALWAYS takes precedence over division, just as addition ALWAYS takes precedence over subtraction, per the PEMDAS method of operation that was cited in my links, and has been taught to me my entire life, with which Big3 also agreed with.
We both cited sources that are completely conflicting with one another as far as the precedence involved within the order of operations goes. One source says A, the other says B. Both are not correct, but both are not wrong either. As MoonlitSunshine has said, the equation is too ambiguous at this point to be correctly solved using either method of operation.
Quote:
Originally Posted by CanwllCorfe
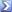
I'm sorry, but I've literally never heard anyone ever say one was more important than the other. I even Googled the problem, and Google calculator was the only thing that came up. It told me the answer was 9.
|
And I have literally NEVER heard anyone say that they bear the same precedence to one another. I was never taught that in my middle school Pre-Algebra class, Statistics, Algebra, Pre-Calculus, Trigonometry, Calculus I, Calculus II, Calculus III, or the Differential Equations courses that I have completed. I feel that my professors who hold Masters and Doctorates degrees would be a better authority than Google. Again, there seems to be a fundamental difference between the sources that really makes no sense to me.
But if asked to explain why one method of operation would be superior to the other, I would posit this:
Addition is positive, meaning that you are adding something to the number. Subtraction is negative, meaning that you are taking something away from the number. Rather than a left to right interpretation for a method of operation between the two, one should use a Positive>Negative order of operation, as a positive number > a negative number. Do the positive (Addition) first, and the negative (Subtraction) second.
Thusly, Multiplication should be considered the "positive" operation, as the exponents are positive, and Division should be considered the "negative" operation, as it is the inverse of multiplacation, meaning that the exponent is negative.
As all sources have stated, exponents takes precedence over ALL other operations. Per the transitive property, Multiplication (utilizing a positive exponent) therefore takes precedence over Division (utilizing in essence a negative exponent).
With that being said, and Multiplication being the positive operation, and Division being the negative operation, it is easy to come to the result that:
6/2*(1+2) = 6/2*(3) = 6/6 = 1





 Threaded Mode
Threaded Mode