Neapolitan, that is impressive. You have again quoted one of my posts, completely failed to read it, and restated your argument.
Mathematics is not always consistent. If it is defined properly and is being used within the bounds that have been shown to be consistent, then yes, it is consistent, but it is not magically always right.
Quote:
Originally Posted by Neapolitan
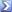
One thing about Mathematics is that it is always consistent, 2+2=4. The fault is not in the numbers or not necessarily the way the problem is written, nor is it the case that it is possible the equation is able to yields two different results. The problem is how one interprets the equation.
|
2+2=4 for a number of reasons, but the easiest axiom to take that proves it is that 2 is defined as 1+1, and 4 as 1+1+1+1, so 2+2 = (1+1)+(1+1) = 4. That's an axiom of addition. However, nowhere in the axioms of addition
Or multiplication does it say "Multiplication and Division are of equal precedence and so
if there is ambiguity do them from left to right to find the proper answer". The simple fact that so many people disagree with this is ample proof by contradiction that there is no one standard convention. How hard is this to see?
Quote:
|
"Schrödinger's Cat" is only a paradox when the box is closed (No **** sherlock, that's the point. Once it is open then the answer is quite clear (so is the answer to the equation when the parentheses are added). Same with the equation, it is only a paradox when someone unaware of proceed with the function of the equation. The conventions in math are not arbitrary and put in place so it's possible the equation can yield two different answers. Once some one understand the rules involved in Algebra there isn't a paradox. If you open the box even Schrödinger's cat will say 6/2*(1+2)=9
|
What you stated about Schrodinger's Cat means either you misunderstood the point I was making, or you are claiming that the Cat will
always be dead or
always be alive when we "open the box". i.e, when parentheses are added (giving the necessary information to find a unique answer) the answer will always be 9. Which is, as I have proven multiple times in this thread, completely wrong.
Quote:
When one takes the problem 6/2*(1+2)
6/2 could be seen as a fraction (as six halves) or the equation 6÷2 in either case the parentheses are not the problem, both will equal "9."
|
Ok, first of all, there is no "fraction" in this equation. The simple act of taking 6/2 to mean the fraction "6 over 2" is the first mistake you're making there. Unless, of course, you want to argue that the convention of using / to represent ÷ is non-standard? Because I'd hate to think that there might be ambiguity in mathematical convention, wouldn't you? Secondly "Both will equal 9"? Again, you're blindly refusing to even consider that there might be a different convention on how this can be solved, and just assuming that your way is right.
Of course if you use your convention, no matter what way you solve it you're going to get 9, that's part of the point I'm making. If you step outside your beloved convention
just for a second, you might see what I'm talking about.
[QUOTE]If one take into consideration it is a fraction, it only yield "1" when one considers 2*(1+2) the denominator as a coefficient of (1+2), and one ignores the numerator as also a coefficient of (1+2). [QUOTE]
I actually have no idea what you're saying here. I'll happily address it if you want to explain what you mean, but I would imagine that it doesn't really affect the overall verity of the post.
Quote:
|
And it one considers 6/2 as 6÷2 then the problem 6÷2(1+2) or 6÷2×3, it will only yield "1" when one does not follow convention and doesn't do operations from the left to the right. One the about Math is that one can tackle a problem six ways to Sunday and still end up with the correct answer provided if one follows proper procedure and convention. If you take consistency and convention out of Math, and make things in it relative you will have 2+2=5.
|
I've bolded the important part in that statement. I must say, congratulations on your impressive level of blind faith in convention. Even when someone states and proves that it might not be standard and infallible, you don't for a second even think about trying to show that it's right. You just keep on using it. What is your justification for assuming that your convention is the right one?
Can you prove your convention is correct from Base Principles? If you can't, then it's not a mathematical constant, it is simply a convention, and a non-standard one at that. A common one, yes, but nowhere near universal. "One thing" about Maths is that when it is taught at 1st and 2nd level, a
lot of shortcuts are taken in order to make it easier to teach. "Rules" are given so that the concept of ambiguity can be avoided, because maths is difficult enough when people are sure that there is one right answer.
Your final statement about 2+2=5... well, I've already addressed that point. Until you can show me an axiom which states the order of operators (even only defined on the Field of Real Numbers), that argument is invalid.
@Tuna:
Quote:
|
Yes, because the parenthesis you showed that Google had on them are unneccessary. They only make it easier for people to see how the process is. Lack of parentheses implies that it's a 6/2 fraction.
|
It does? Really? That's the first time I've heard that particular convention. Can you show me where it states that that's the case, in the axioms of multiplication?
I would like to remind anyone still arguing this that I'm not saying that your answer is wrong. By all means, it's the most popular way of solving the equation. It's just not Syntactically correct, which means that even though the most common convention states that the first pair of parentheses can be assumed around (6/2), it is an assumption, and so long as they are not there, you can claim that the = 1 answer is just as correct.
@Freebase: That's part of what I'm saying, certainly. It's more that nothing in maths can be taken to be certain unless it is defined that way, given as an axiom (a statement so obvious that it cannot be proven), or provable from the definitions and axioms. Nowhere in any of the axioms that I have been taught regarding the Real Numbers (the number line, essentially) is there an axiom or a definition which states the universal order of priority of operations. There is a standard, which is
almost universal, but as is evident from this thread, not entirely so. That's what my point boils down to, that a convention that is not a definition, not universal and not provable from the axioms is being taken as irrefutably true.
@CanwllCorfe: I understand your belief, but I'm afraid that it's as a result of the way maths is taught: Certainly among at least 50% of the population of at least the western world, you would be safe in making such assumptions, but mathematics is not a subject where you can say "because it holds for the majority, it holds for all". Indeed, there are still some theorems out there that remain unproven even though we know that it holds for every case that we can possibly calculate, just because we can't prove it for a general case. Does that make sense?



 Linear Mode
Linear Mode
